
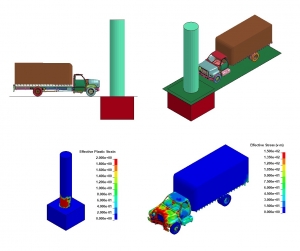

Performance of bridge piers under vehicle collision
- Vehicle collision
- Impact load
- Bridge column
- Design force
- Ls-Dyna
Both the peak dynamic force (PDF) and the equivalent static force (ESF) of a vehicle collision with reinforced concrete bridge columns were examined as part of an extensive finite element (FE) analyses study. An extensive parametric study of 13 parameters, including the concrete material model, the unconfined concrete compressive strength , the material strain rate, the percentage of longitudinal reinforcement, the hoop reinforcement, the column span-to-depth ratio, the column diameter, the top boundary conditions, the axial load level, the vehicle’s velocity, the vehicle’s mass, the roadside distance between errant vehicle and unshielded bridge column, and the soil depth above the top of the column footing was conducted. Three approaches were used to investigate the ESF. The ESF in the first (stiffness-based) approach was defined as the static force producing the same maximum displacement that is produced by a vehicle collision at the point of impact. The ESF examined in the second approach was calculated according to the Eurocode. The ESF studied in the third approach was defined as the Peak of the Twenty-five Milli Second moving Average (PTMSA). The different ESFs were compared to the ESF in the American Association of State Highway and Transportation Officials-Load and Resistance Factor Design (AASHTO-LRFD; 2670 kN [600 kips]). In general, the ESF calculated according to the Eurocode presented the lower bound while those from the stiffness-based approach presented the upper bound. Furthermore, the recommended ESF of the AASHTO-LRFD was found to be non-conservative for heavy and/or high speed vehicle impacts; it was found to be too conservative for light and/or slow vehicle impacts. Hence, rather than a constant design impact force, a variable design impact force should be used. An equation was developed to calculate a design impact force, which is the function in the vehicle’s mass and velocity. A simplified equation based on the Eurocode equation of the ESF was proposed. These equations, however, do not require cumbersome FE analyses.
Our services
Consulting and conducting industrial and academic projects related to mechanical engineering, civil engineering, biomedical engineering, mining engineering, etc. based on Finite Element Modeling (FEM)
Contact
Just feel free and let us know if you have any questions and we are looking forward to hearing from you guys.
Email: info@rezarazaghi.com
WhatsApp: +989397091737


